DPL

Whatever you want to call them, the focus of this research is the formation of singularities on the free surface of a liquid (see our publications below). A free surface of a fluid undergoing Faraday excitation exhibits highly focused surface waves which form thin fluid filaments, or jets, that can eject droplets and spray from their tips. We are exploring the mechanism by which such singularities are formed.

We excite a cylindrical tank partially filled with a glycerin-water mixture with a sinusoidal forcing amplitude. Below a threshold amplitude, periodic wave states dominate, while the singularities occur in aperiodic states at higher amplitudes. By modulating the acceleration amplitude at a fixed frequency, we produce a repeatable singularity for investigation. We use high-speed video and high-resolution stroboscopic photography to explore the dynamics of the singularity.

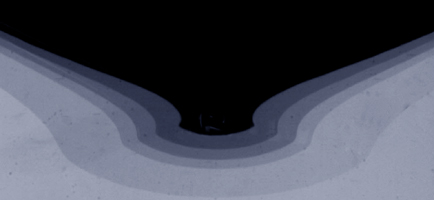
When a tall, narrow wave collapses, it creates a roughly cylindrical depression on the surface of the fluid. The collapse of the walls of this depression focuses energy along the central axis of the tank and forces the fluid upward very quickly, forming the filament. We found that an important factor in the production of the singularities was the height of the last smooth standing wave before the singularity. The collapse of this wave produces the depression which collapses to produce the jet, and three types of depressions occur (see image below). Below a certain critical wave height, the collapsing depression is smooth with no overhang. Above this height, however, the surface pinches off and a bubble is entrained.  The entrainment of the bubble greatly reduces the energy focused in the jet.  For a collapsing wave of the critical height, the collapse occurs at the cusp of a topology change and the energy is maximally focused.  We have measure jet tip velocities of over 80 m/s!

We capture a time sequence of images of the collapse of the surface profile into a singularity. Such images offer a means by which we can test a similarity solution for the profile for times preceding the singularity of the form:

h=( to-t )α f( r( to-t )β ),

where h and r are the height and radius of the surface profile, to is the time of the singularity, f is a scaling function, and α and β are parameters determined by the Bernoulli equation, the incompressibility condition, and the kinetic equation for the free surface. We have studied a similarity solution for the collapse of surface profiles after the singularity. Theoretical work suggest uniquely determined values of α and β in the similarity solution, and we have established from experimental work that the surface does indeed undergo a self-similar collapse as expected.  Additionally, we have calculated a numerical solution to the equations of motion for the self-similar collapse which is in good agreement with the experimental results.  This work was recently published in a letter to Nature.

From this study, we hope to better understand ocean waves and the air-ocean interaction, as well as guide the applied mathematics of singularity formation in nonlinear systems.

Publications